To introduce you to Maple, we'll use it to analyze the problem of projectile motion in two dimensions. However, before we can tell Maple what to do, it is essential that we understand the problem.
Suppose our projectile is fired at time t=0 from the initial
position ![]() . The initial speed is
. The initial speed is ![]() and the
projectile is fired at an angle
and the
projectile is fired at an angle ![]() with the horizontal. If air
resistance is ignored, Newton's second law can be used to derive the
following equations for the position (x(t),y(t)) of the projectile
at time t. The letter g is used to stand for the acceleration due
to gravity.
with the horizontal. If air
resistance is ignored, Newton's second law can be used to derive the
following equations for the position (x(t),y(t)) of the projectile
at time t. The letter g is used to stand for the acceleration due
to gravity.
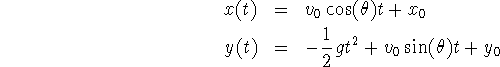
In these equations, t is the independent variable, x and y are
the dependent variables, and g, ![]() ,
, ![]() ,
, ![]() , and
, and ![]() are parameters. That is, we think of g,
are parameters. That is, we think of g, ![]() ,
, ![]() ,
, ![]() , and
, and
![]() as being constants. Once we have set values for the constants,
then the equations give us the x and y coordinates of the
projectile as functions of t.
as being constants. Once we have set values for the constants,
then the equations give us the x and y coordinates of the
projectile as functions of t.
Here are some examples of the kinds of questions that these equations can be used to answer.