Exercises

1.

Give an example of a situations where the D operator would be easier to use than the diff command. Then give an example where the diff command would be better. In each case, make sure you justify your choice.

2.

Consider the function

$$g(x) = \frac{{x}^{4}- 8.0{x}^{3}- 74.59{x}^{2}+ 279.95x+ 469.56}{x^2+2.15}$$

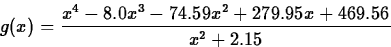
Find all the values of x for which the derivative of g is zero.

3.

The tangent line to a function at a particular value of x intersects the graph of the function at least once, at the point of tangency. However, the tangent line may intersect the graph at other points. In this problem, we investigate whether the tangent line at one point can also be tangent to the graph at another point. For example, consider the function

g(x) = (x²-1)²

You should be able to show that the tangent line at x=-1 is also tangent to the graph at x=1. Next, suppose we change the function slightly.

h(x) = (x²-1)² +x/2

Is it still possible to find two different values of x such that the tangent lines coincide? The answer is yes. Find them.