Exercises

1.

Consider 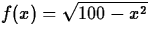$f(x) = \sqrt{100-x^2}$ on the interval [0,10].

(a)

For n = 8,16, and 32, approximate the area under the curve by using each of the left endpoint rule, right endpoint rule and midpoint rule.

(b)

Use your knowledge of geometry to obtain the exact value of the area. Which of the three rules seems to give the best approximation to the area for a given n? Explain.

(c)

What is the smallest n for which the midpoint rule approximation is accurate to two decimal places, i.e, that 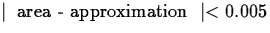$\mid \mbox{ area - approximation } \mid < 0.005$? Justify your answer by showing that your n does work and that n - 1 does not work. Comment as needed on your work.

(d)

Check the accuracy of the left endpoint and right endpoint rules when n = 9900. Does either one give an approximation with 2-place accuracy? Which of the three rules seems to be better? Why?

2.

Consider $g(x) = \displaystyle\frac{x^2 + 1}{x^2 - 1}$ on the interval [2,5].

(a)

Use the appropriate box command with 9 rectangles to display an upper sum approximation to the area under the curve. Explain your choice of box command.

(b)

For n = 20,40 and 80, approximate the area under the curve with an upper sum.

(c)

Use the appropriate box command with 9 rectangles to display a lower sum approximation to the area under the curve. Explain your choice of box command.

(d)

For n = 20,40,80, approximate the area under the curve with a lower sum.

(e)

Find the smallest value of n for which the upper sum minus the lower sum will be less than 0.005. Justify your answer by showing that your n does work and that n - 1 does not work. Comment as needed on your work.

(f)

Based on your answer in (e), give the interval in which the actual value of the area must lie. Explain.

3.

Consider h(x) = 3 + 2x - x² on the inteval [0,3]. For n = 60, find the upper sum and lower sum approximations to the area under the curve. Be sure to indicate clearly which is which and to comment on the motivation for your use of the expressions you evaluate. (Be careful; you may want to use box commands to picture what you are doing. Also, be careful as to how you treat the subintervals.)

4.

Consider k(x) = x³ - 2x² - 5x + 6. We discuss the total square units of area bounded by the graph of the function and the x-axis on the interval [1,4].

(a)

For n = 60, do a midpoint rule approximation. Note that k(3.5) = 6.875 and k(4) = 18. Explain why this suggests there are more than 3 square units of area.

(b)

Note the apparent contradiction implicit in (a) and explain how to resolve it.

(c)

For n = 60, find a true midpoint rule approximation to the total square units of area.

5.

Previously we have considered the area between the graph of a function and the x-axis. Now we look at the area between two graphs. Consider f(x) = x³ + 1 and g(x) = x² - x + 1 on the interval [0,1]. For n = 80, do a midpoint rule approximation to the area between the graphs of these two functions. Discuss how you are describing the heights of the approximating rectangles.