Fermat's Principle and the Brachistochrone
Fermat's Principle and Snell's Law
It's gratifying to know that simple observations we make everyday can be explained using calculus. For example, a stick which is partly dipped into water looks broken. One conclusion we can draw from this observation is that light sharply changes its direction as it crosses the interface between two different media. So, if light goes from some point P in water to a point Q in the air (see Fig. 1), its path is bent so that it doesn't follow a straight line. Unfortunately, this violates the assumption that light chooses the path of shortest distance.
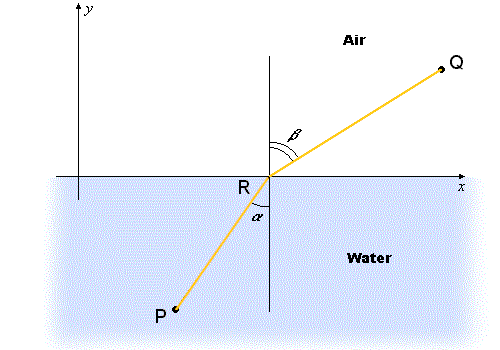
Figure 1: Refraction of light
The French mathematician Pierre de Fermat instead suggested another principle called the principle of least time. Fermat conjectured that of all the paths that light might choose to get from one point to another, it always chooses the path which takes the least amount of time. This principle is more general since it equally applies to both uniform and non-uniform media. Various media differ by the velocity at which light propagates through them. For example, in water, the velocity of light equals cW = 225,056 km/sec while in the air it equals cA = 291,262 km/sec. Remembering the formula rate.time = distance, according to Fermat's Principle, light will choose the path that minimizes the quantity
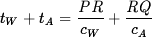 | (1) |
which is the sum of the two times. If
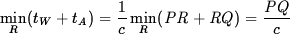
which shows that the path is a straight line as we would expect.
Getting back to Eq. 1, we can assign coordinates

Problem 1
Show that the position

In words, this says that the sine of the angle of incidence  is related to the sine of the angle of refraction
is related to the sine of the angle of refraction 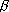 just as the velocity of light in the primary media (water) is related to the velocity of light in the secondary media (air). This relation is known as Snell's Law.
just as the velocity of light in the primary media (water) is related to the velocity of light in the secondary media (air). This relation is known as Snell's Law.
The Brachistochrone
We will apply Snell's Law to the investigation of a famous problem suggested in 1690 by Johann Bernoulli. This problem gave birth to the Calculus of Variations - a powerful branch of mathematics. Bernoulli's problem is as follows: Suppose we have a heavy particle such as a steel ball which starts off at rest at point A. It then slides downward, under the force of gravity alone, along a frictionless track in a vertical plane along some curve through A and another fixed point B. The question is this: What shape should we make the curve if we want to minimize the time of the object's descent from A to B? The curve possessing this property is called a brachistochrone ( ).
).
Johann Bernoulli gave an exclusively beautiful solution of this problem. He knew from physics that a heavy particle will have a speed of
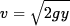 | (2) |
at the vertical distance y measured downward from the particle's original location (
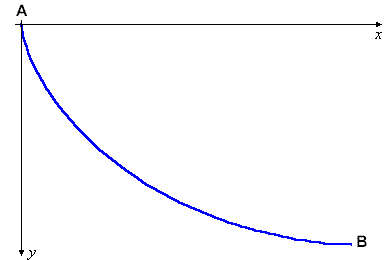
Figure 2: A brachistochrone
Let's split the vertical plane through the curve AB into a series of thin horizontal layers. The speed v can then be assumed to be constant within every layer, but this constant will vary as we move from one layer to another. Where did we encounter a similar situation? We saw something similar in the phenomenon of the refraction of sunlight that passes though a medium which varies from layer to layer. With this in mind, we can look at the curve AB differently. By Fermat's Principle, we can treat this curve as the trajectory of light which passes through an optically nonhomogeneous medium. In short, the light trajectory is a brachistochrone.
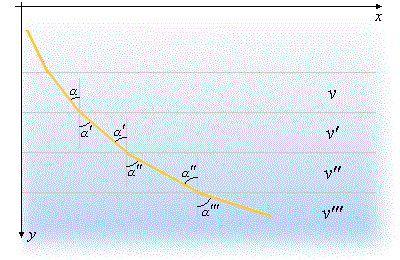
Figure 3: The trajectory of light through a nonhomogeneous medium
If we introduce a large number of layers, each with its own constant value of v, then we arrive at a number of uniform media differing only in the values of the velocity v of light. Within each layer, light will propagate along a straight line, but these lines will change direction at every interface.
If we now apply Snell's Law at each of these interfaces (see
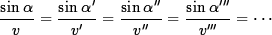
In other words, the ratio
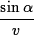
is exactly the same for all layers. If we consider infinitely many layers, this statement still remains valid and, in the limit, we obtain
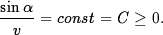 | (3) |
We conclude that the unknown light path (i.e. the particle's trajectory) is governed by
Problem Two
Assume that 
| (4) |
After you construct
 - sin - sin  ) )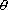 ) )
|
Note: You don't have to determine the constant a (e.g. in terms of other variables).
This curve is called a cycloid. It represents the path a reflector makes if it is attached to a point on the rim of a bicycle wheel (
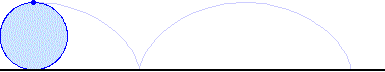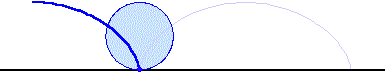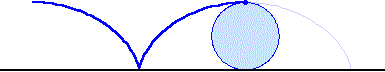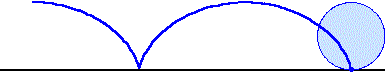
Figure 4: A cycloid
![]() Back to the top
Back to the top
Created by Henry Fink
Last updated: Monday, October 6, 1997