


Next: About this document ...
Up: lab_template
Previous: lab_template
Subsections
The purpose of this lab is to acquaint you with the Maple commands for triple integrals.
To assist you, there is a worksheet associated with this lab that contains examples. You can copy that worksheet to your home directory with the following command which must be run in a terminal window (such as teraterm), not in Maple.
cp /math/calclab/MA1024/tripleint.mws My_Documents
You can copy the worksheet now, but you should read through the lab before you load it into Maple. Once you have read to the exercises, start Maple, load the worksheet, and go through it carefully. Then you can begin the exercises.
In double integrals we saw how to calculate volume by integrating a three dimensional function using limits of intgration of a bounded AREA. In triple integrals, the integral will be taken of a four-dimensional function using limits of integration of a bounded VOLUME.
In doulble integrals the three-dimensional function could be pictured as well as the two-dimensional domain. In triple integrals, the four-dimensional function cannot be visualized but the three-dimensional domain can be. Remember this, with triple integrals the plot represents the limits of integration.
- Find the volume of the cylinder
 bounded by the planes
bounded by the planes  and
and  in Cartesian coordinates by
in Cartesian coordinates by
- a)
- integrating in the order
 .
.
- b)
- integrating in the order
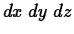
- Find the value of the triple integral of
given the domain as the volume bounded by the plane  and
and
 .
.



Next: About this document ...
Up: lab_template
Previous: lab_template
Jane E Bouchard
2005-12-05

