- a.
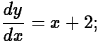 y(2) = 3.
Integrating,
y(2) = 3.
Integrating,
Substituting in the initial value,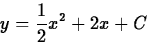
The solution is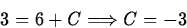
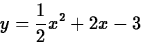
- b.
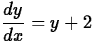 ; y(2) = 3.
The general solution is
; y(2) = 3.
The general solution is
y = -2 + Cex
Substituting in the initial value,
The solution is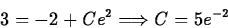
y = -2 + 5ex-2
![]()
- a.
- Find a nontrivial solution to the homogeneous equation.
The homogeneous equation is x' = 4x; therefore
xh = e4t
is a homogeneous solution. - b.
- Find a particular solution to the differential equation
Try to find a solution of the form
Substituting,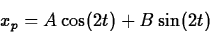
Grouping sines and cosines,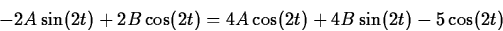
Solving gives A = 1 and B = -1/2. Therefore
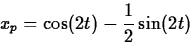
- c.
- Find the general solution to the equation.
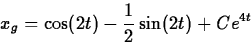
- d.
- Consider the solutions with two different initial
values. Do the solutions get farther apart or closer together as t
increases? Justify your answer.
The solutions will be
for two different constants C1 and C2.
Their difference is
x2(t)-x1(t) = (C2-C1)xh(t) = (C2-C1)e4t
Since the exponential gets large as t gets large, the solutions get further apart.
- a.
- Write an expression for the amount of water that is
added to the tank in a small time period. Write an
expression for the amount of water that leaves the tank
in the same small time period.
Define any variables you use.
Define:
 :
:- length of small time period
- V:
- volume of water
- k:
- proportionality constant between leakage and volume (leakage rate = kV)
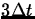 .
.
Amount lost is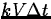 .
.But initially, V = 400 and the rate of leakage is 4, so k = 0.01.
Amount added is
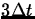 .
.
Amount lost is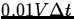 .
. - b.
- Write an equation that gives the change in the amount
of water over a small time period. Use this equation
to create a differential equation model for the amount
of water in the tank as a function of time.
Change over
 is
is
The differential equation is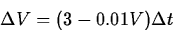
Including the initial value, the model is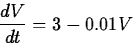
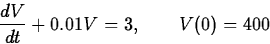
- c.
- What is the physical meaning of the free and
forced response of this model?
The free response solves
V' + 0.01V = 0; V(0) = 400
It is the amount of water there would be if you started with 400 liters, added none, and allowed the water to leak out as before.The forced response solves
V' + 0.01V = 3; V(0) = 400
It is the amount of water there would be if you started with an empty tank, added water as in the original problem, and allowed water to leak out as in the original problem.
- a.
- Find the equilibria of this differential equation.
Solve -2 - u + u2 = 0; the equilibrium are
at u = 2 and u=-1
- b.
- Where are solutions to this equation increasing?
Where are they decreasing?
If u>2, then -2-u+u2>0.
If -1<u<2, then -2-u+u2<0.
If u<-1, then -2-u+u2>0.
The solution is increasing if u>2 or u<-1. It is decreasing if -1<u<2.
- c.
- Decide whether each equilibrium in part (a) is stable
or unstable.
The equilibrium at -1 is stable. The equilibrium at 2 is unstable.