- a.
- What is the fitted regression line?
Interpret the fitted slope. Is it
reasonable to interpret the intercept?
If so, interpret it. If not, tell why not.
Answer:
The change in predicted LOG10EFF is 0.0067 per year. (5 points) The intercept is well beyond the range of the data, and as such it is not reasonable to interpret it. In fact, it is not clear what steam technology, as we know it, existed in the year 0. (5 points)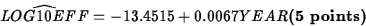
- b.
- By what percentage does use of this model
reduce the uncertainty in predicting the log of efficiency?
Answer: 99.26% (10 points)
- c.
- What is the value of the Pearson correlation
coefficient between the log of efficiency and year?
Answer: 0.9963 (10 points)
- d.
- Estimate
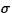 , the standard deviation
of the random errors.
, the standard deviation
of the random errors.
Answer:
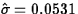 (10 points)
(10 points)
- e.
- The standard deviation of the 11 values of
YEAR in the data set is 86.4875. Use this, the output
in Figure 1, and your knowledge of
regression to obtain the standard deviation of the 11
values of LOG10EFF in the data set.
Answer: We know that
Therefore, SY=0.5816. (5 points)
- (a)
- Summarize these data using overall, row and
column percentages.
Frequency Percent Row Pct Col Pct Boys Girls Total Left-handed 9 11 20 11.11 13.58 24.69 45.00 55.00 23.68 25.58 Right-handed 29 32 61 35.80 39.51 75.31 47.54 52.46 76.32 74.42 Total 38 43 81 46.91 53.09 100.00 - (b)
- What are the marginal distributions of gender
and handedness?
Gender: 46.91% boys, 53.09% girls. Handedness: 24.69% left, 75.31% right.
- (c)
- What is the conditional distribution of
handedness given the individual is male? Female?
Females: 25.58% left, 74.42% right. Males: 23.68% left, 76.32% right.
- (d)
- Compute the Pearson residual in each cell
under the assumption that gender and handedness are
independent. Do any cells have perticularly large
Pearson residual values?
For the 1,1 cell, the expected frequency is
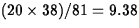 , and the Pearson residual is
, and the Pearson residual is
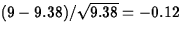 . The Pearson residual for the 1,2 cell is
0.12, for the 2,1 cell is 0.07, and for the 2,2 cell is
-0.07. None of these values is large.
. The Pearson residual for the 1,2 cell is
0.12, for the 2,1 cell is 0.07, and for the 2,2 cell is
-0.07. None of these values is large.
- (e)
- Conduct a
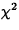 test for the independence
of gender and handedness at the 0.05 level of
significance. What do you conclude?
test for the independence
of gender and handedness at the 0.05 level of
significance. What do you conclude?
The value of the
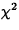 test statistic is
-0.122+0.072+0.122+(-0.072)=0.0386, which has a p-value of
0.843 (Or, note that 0.0386 is less than
test statistic is
-0.122+0.072+0.122+(-0.072)=0.0386, which has a p-value of
0.843 (Or, note that 0.0386 is less than 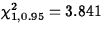 ).
So there is little evidence of a relation between gender and handedness.
).
So there is little evidence of a relation between gender and handedness.
![\begin{figure}
\centerline{\includegraphics*[height=9in,width=5in]{oldt2fig1.eps}}
\vspace{2ex}\end{figure}](img5.gif)