


Next: About this document ...
Up: lab_template
Previous: lab_template
Subsections
The purpose of this lab is to use Maple to study applications of
exponential and logarithmic functions. These are used to model many
types of growth and decay.
The simple model for growth is exponential growth, where
it is assumed that
 is proportional to
is proportional to 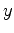 . That is,
. That is,
Separating the variables and integrating (see section 4.4 of the text),
we have
so that
In the case of exponential growth, we can drop the absolute value
signs around 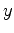 , because
, because 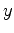 will always be a positive quantity.
Solving for
will always be a positive quantity.
Solving for 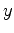 , we obtain
, we obtain
which we may write in the form 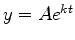 , where
, where 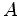 is an
arbitrary positive constant.
is an
arbitrary positive constant.
In a sample of a radioactive material, the
rate at which atoms decay is proportional to the amount of material present.
That is,
where 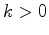 is a constant. This is the same equation as in exponential growth,
except that
is a constant. This is the same equation as in exponential growth,
except that  replaces
replaces 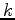 . The solution is
. The solution is
where 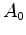 is a positive constant. Physically,
is a positive constant. Physically, 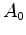 is the amount of
material present at
is the amount of
material present at 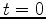 .
.
Radioactivity is often expressed in terms of an element's half-life.
For example, the half-life of carbon-14 is 5730 years. This statement means
that for any given sample of
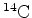 , after 5730 years, half of it
will have undergone decay.
So, if the half-life is of an element Z is
, after 5730 years, half of it
will have undergone decay.
So, if the half-life is of an element Z is 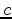 years, it must be
that
years, it must be
that
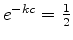 , so that
, so that 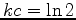 and
and
 .
.
What is usually called Newton's law of cooling is a simple model for
the change in temperature of an object that is in contact with an
environment at a different temperature. It says that the rate of
change of the temperature of an object is proportional to the
difference between the object's temperature and the temperature of the
environment. Mathematically, this can be expressed as the differential
equation
where 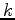 is the constant of proportionality and
is the constant of proportionality and
 is
the temperature of the environment. Using a technique called
separation of variables it isn't hard to derive the solution
is
the temperature of the environment. Using a technique called
separation of variables it isn't hard to derive the solution
where 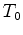 is the temperature of the object at
is the temperature of the object at 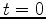 .
.
Information can be thought of as of a physical quantity which can be measured. According to the Gallup Institute, information news diffuses through a fixed adult population of size 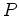 at a rate of time proportional to the number of people who have not heard the news.
at a rate of time proportional to the number of people who have not heard the news.
If 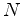 is the number of people who have heard the news after t days, then
is the number of people who have heard the news after t days, then
The initial condition  yields the solution
yields the solution
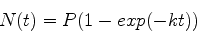
- Suppose that the population of a certain bacteria can be modeled by an exponential function. In a particular experiment, the number of bacteria was
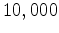 at
at 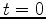 . Four hours later, the bacteria was
. Four hours later, the bacteria was 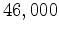 . Find the value of the growth constant
. Find the value of the growth constant 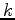 and use it to predict the number of bacteria that would have been present after
and use it to predict the number of bacteria that would have been present after 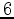 hours.
hours.
- The worst nuclear accident in history happened in 1986 at the Chernobyl nuclear plant near Kiev in the Ukraine. An explosion destroyed one of the plant's four reactors, realeasing large amount of radioactive isotopes into the atmosphere. Consider 10 grams of the plutonium isotope Pu-239 released in the Chernobyl nuclear accident. This isotope has a half-life of
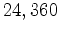 years. How long will it take for the 10 grams to decay to 1 gram?
years. How long will it take for the 10 grams to decay to 1 gram?
- A thermometer registered
 outside and then was brought into the house where the temperature was
outside and then was brought into the house where the temperature was
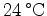 . After 5 minutes, it registered
. After 5 minutes, it registered
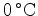 . When will it register
. When will it register
 ?
?
- Suppose that 75% of a freshman class of 500 students on a campus heard about the market crash on Wall Street 3 days after it happened. How long will it take for 99% of the freshman to hear the news?



Next: About this document ...
Up: lab_template
Previous: lab_template
Dina J. Solitro-Rassias
2010-10-07
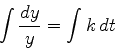
![]() , after 5730 years, half of it
will have undergone decay.
So, if the half-life is of an element Z is
, after 5730 years, half of it
will have undergone decay.
So, if the half-life is of an element Z is ![]() years, it must be
that
years, it must be
that
![]() , so that
, so that ![]() and
and
![]() .
.
![]() is the number of people who have heard the news after t days, then
is the number of people who have heard the news after t days, then
![]() yields the solution
yields the solution