Exponentials and Logarithms

When the exponential function 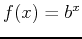$f(x)=b^x$ was introduced, (for 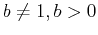$b\neq1,b>0$) you saw that the function is increasing if 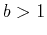$b>1$ and decreasing if 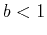$b<1$. You can observe the monotonicity by plotting 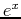$e^x$ and $0.1^x$.

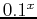> plot(exp(x),x=-1..1);
> plot(0.1^x,x=-1..1);

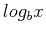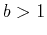The logarithmic function $log_{b}x$ was introduced for $b>0,b\neq1$ as the inverse of the exponential. The logarithm is therefore inreasing if $b>1$ and decreasing if 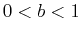$0<b<1$. Indeed you can plot 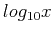$log_{10}x$ and $log_{0.1}x$.

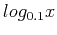> plot(log[10](x),x=0.1..10);
> plot(log[0.1](x),x=0.1..10);

From the monotonicity properties you can compare two logarithms having the same base without computing their values:

$$log_{5}10<log_{5}12$$

$$log_{1/2}9<log_{1/2}6$$

We can also see what is the behavior of the logarithm of different bases by using the 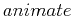$animate$ command. To get the animation to play, just click on the graph and click on the go button in the tool bar.

> with(plots):
> animate(log[b](x),x=0.1..10,b=1.1..10,frames=30);
> animate(log[b](x),x=0.1..10,b=0.01..0.1,frames=30);

From the monotonicuty properties you can see that for a fixed 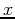$x$ in the interval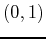$(0,1)$ the logarithm increases with 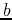$b$ but for 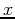$x$ in the interval 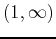$(1,\infty)$ it decreases. As a result you can now compare logarithms with different bases without computing their values.

$$log_{3}7<log_{2}7$$

$$log_{1/2}5<log_{1/3}5$$

Here are some examples using Maple to solve logarithmic and exponential equations.

$$5^{x+1}+5^x+5^{x-1}=155$$

$$log_{5}(2x^2+2x+5)=2$$

> solve(5^(x+1)+5^x+5^(x-1)=155,x);

> solve(log[5](2*x^2+2*x+5)=2,x);