


Next: About this document ...
Up: lab_template
Previous: lab_template
Subsections
The purpose of this lab is to acquaint you with using Maple to do
double integrals.
To assist you, there is a worksheet associated with this lab that
contains examples and even solutions to some of the exercises. You can
copy that worksheet to your home directory by going to your computer's Start menu and choose run. In the run field type:
\\filer\calclab
when you hit enter, you can then choose MA1024 and then choose the worksheet
Doubleint_start_C12.mw
Remember to immediately save it in your own home directory. Once you've copied and saved the worksheet, read through the background on the internet and the background of the worksheet before starting the exercises.
Suppose that  is a rectangular region in the the
is a rectangular region in the the 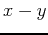 plane, and
that
plane, and
that 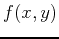 is a continuous, non-negative function on
is a continuous, non-negative function on  . Then the
volume of the solid above
. Then the
volume of the solid above  and below
and below 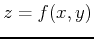 is given by the
double integral
is given by the
double integral
You learned in class that such integrals can be evaluated by either of
the iterated integrals
or
where the rectangle  is defined by the inequalities
is defined by the inequalities
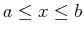 and
and
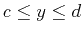 .
.
The worksheet associated with this lab contains examples of how to use
Maple to compute double integrals. It also has an example of how to
use Maple if the region of integration is of the more complicated form
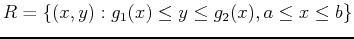 .
This is the case where the base of the solid is not rectangular, but
is bounded by two curves
.
This is the case where the base of the solid is not rectangular, but
is bounded by two curves 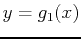 and
and 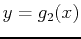 . If
. If 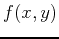 is as
before, then the volume of the solid above
is as
before, then the volume of the solid above 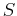 and below
and below 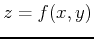 is
given by
is
given by
The other case, where the region  is
is 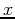 -simple can also be handled
using Maple, and there is an example in the worksheet.
-simple can also be handled
using Maple, and there is an example in the worksheet.
- Use Maple to compute the following double integrals.
- a)
-
- b)
-
- c)
-
- Let
 be the region in the
be the region in the 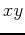 plane bounded by the two curves
plane bounded by the two curves
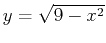 and
and
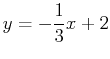 . Use a double integral to compute the area of the region. Use both orders of integration. Include a plot of the region
. Use a double integral to compute the area of the region. Use both orders of integration. Include a plot of the region  in your worksheet.
in your worksheet.
- Use a double integral to find the volume of the region bounded by the two paraboloids
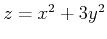 and
and 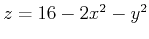 .
.
- Use a double integral to prove that the volume of a cylinder of height
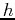 radius
radius 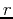 is
is
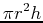 .
.



Next: About this document ...
Up: lab_template
Previous: lab_template
Dina J. Solitro-Rassias
2012-01-29
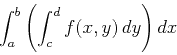
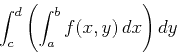
![]() .
This is the case where the base of the solid is not rectangular, but
is bounded by two curves
.
This is the case where the base of the solid is not rectangular, but
is bounded by two curves ![]() and
and ![]() . If
. If ![]() is as
before, then the volume of the solid above
is as
before, then the volume of the solid above ![]() and below
and below ![]() is
given by
is
given by
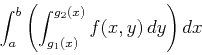
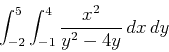
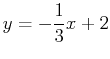 . Use a double integral to compute the area of the region. Use both orders of integration. Include a plot of the region
. Use a double integral to compute the area of the region. Use both orders of integration. Include a plot of the region